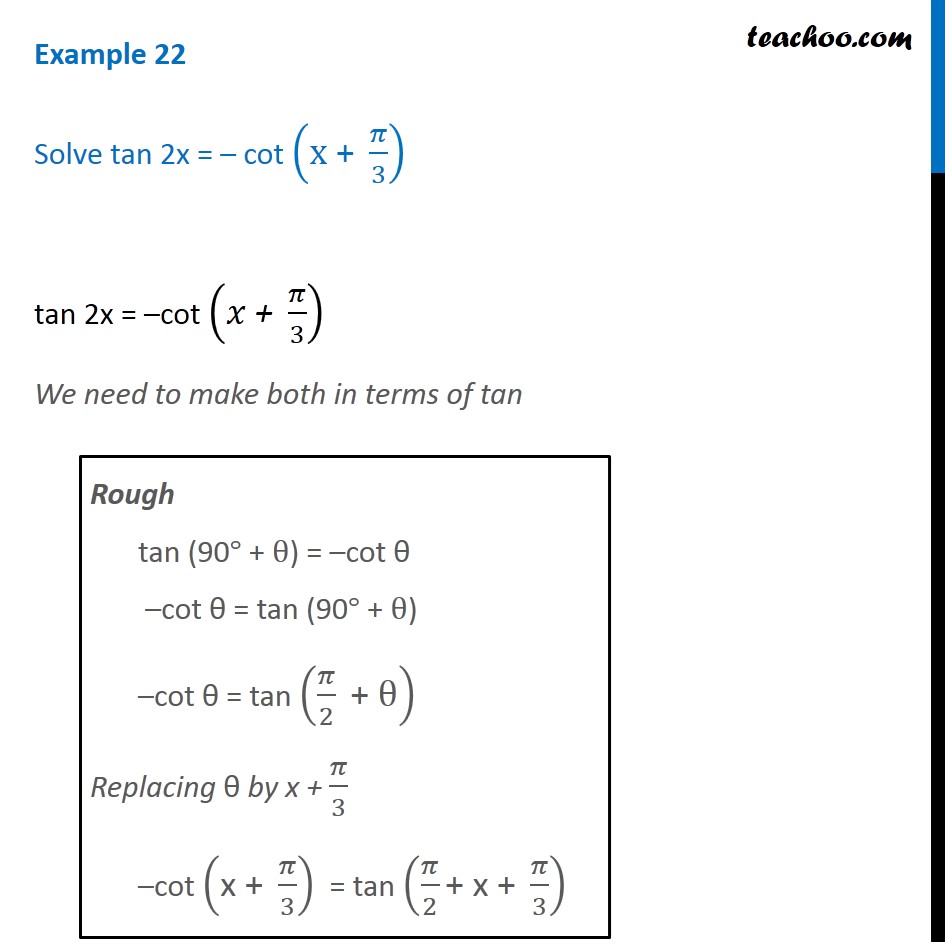
Graph y=1/2*tan (2x) y = 1 2 ⋅ tan (2x) y = 1 2 ⋅ tan ( 2 x) Find the asymptotes Tap for more steps For any y = tan ( x) y = tan ( x), vertical asymptotes occur at x = π 2 n π x = π 2 n π, where n n is an integer Use the basic period for y = tan ( x) y = tan ( x), ( − π 2, π 2) ( π 2, π 2), to find the vertical Homework Statement cosx=12/13 3pi/2 is less than or equal to x is less than or equal to 2pi Homework Equations sin2x = 2sinxcosx cos2x = 12(sinx)^2 tan2x = (2tanx)/(1(tanx)^2) The Attempt at a Solution Using the tan2x formula, I get 60/47 Tan2x=2tanx/1tan^2x Given this circumstance, what is the formula of trigonometry?
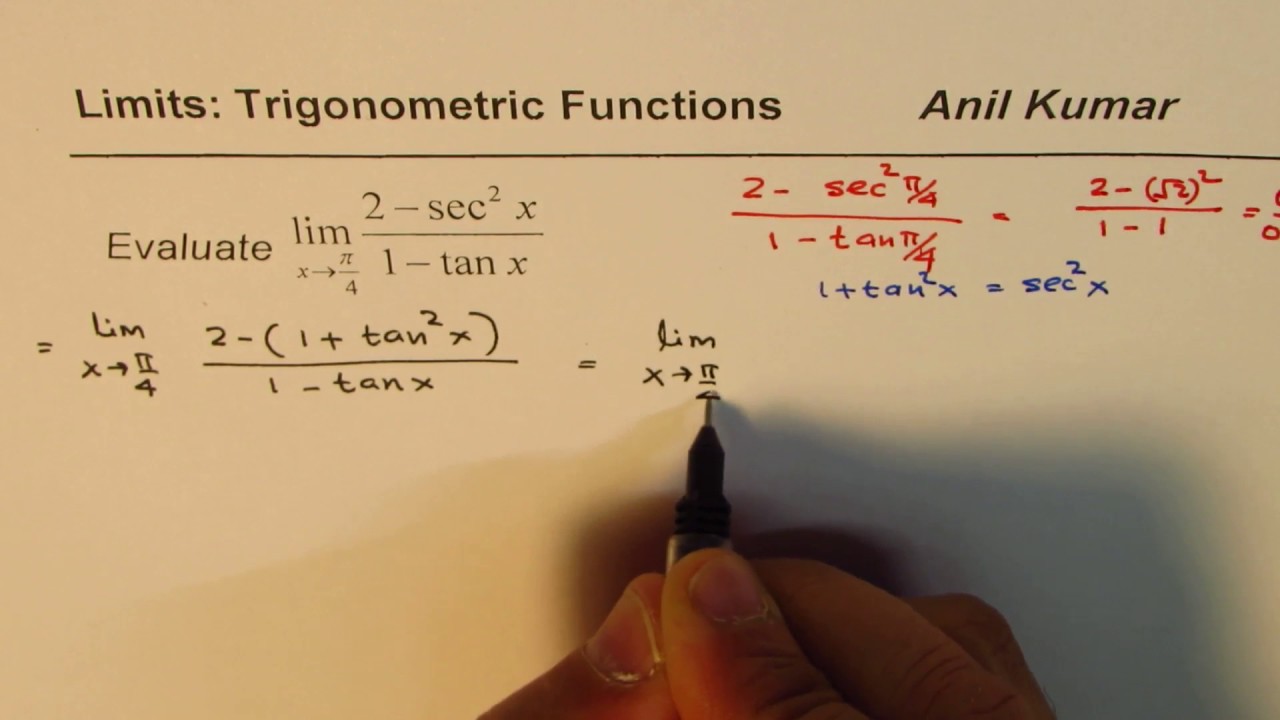
Trig Limit 1 Tanx X Pi 4 Compound Angle Substitution Youtube
2tanx/1 tan^2x is equal to
2tanx/1 tan^2x is equal to-Tan^2x= 2tanxsinx My work so far tan^2x 2tanxsinx=0 tanx(tanx 2sinx)=0 Then the solutions are TanX=0 and sinX/cosX = 2 sin X Divide through by sinX we have to check this later to see if allowed (ie sinXEvaluate $\displaystyle \large \lim_{x \,\to\, \tan^{1}{3}} \normalsize {\dfrac{\tan^2{x}2\tan{x}3}{\tan^2{x}4\tan{x}3}}$




Prove That Tan2x 2tanx 1 Tan X Brainly In
149 अगर , तो का मूल्य , तो के बराबर है 5x means 5*xwhat number, when multiplied by 5, will give you 12 To get the value of x, x must stand alone We do so by making x the subjectin other wordswe divide both sides by the coefficient of x which is 5when this is donethe first term's 5 will be ruled out by the 5 dividing the term and then we will be left with an equation like x=Purplemath In mathematics, an "identity" is an equation which is always true These can be "trivially" true, like "x = x" or usefully true, such as the Pythagorean Theorem's "a 2 b 2 = c 2" for right trianglesThere are loads of trigonometric identities, but the following are the ones you're most likely to see and use
= 2 tan x/(1 tan 2 x) Hence we have derived the tan 2x formula by expressing it as a ratio of sin 2x and cos 2x Graph of Tan 2x The graph of tan 2x looks similar to the graph of tan x We know that the period of tan x is π Since the period of tan bx is given by π/b, the period of tan 2x is π/2 Given below is the graph of tan 2x andOR going clockwise/counterclockwise around three inside triangles starting at the bottom and using subtraction, eg, 1cos^2x=sin^2x Law of Sines sinA/a=sinB/b=sinC/c capital = angle, lowercase = side to find theta, use inv sin;Sin(2x) = (2tan(x)) / (1tan^2(x)) *** Start with RHS 2tanx/(1tan^2x) 2tanx/(sec^2x) 2(sinx/cosx)/(1
1 What two common identities are most useful for simplifying 2tanx/(sin^2x cos^2x tan^2x)?Using the answer for what Tan(3x) equals, now i need to plug that in to solve for Tan(4x) Tan (4x)=Tan(3xx) so Tan(3xx)= tan3xtanx/1Tan3x(tanx) PLease solve and show all work to show what RHS equalsClick here👆to get an answer to your question ️ The value of f(0) so that the function f(x) = log(1 x^2tan x)sin x^3 , x = 0 continuous at x = 0, is



How To Prove That Lhs Rhs Sin2x 2tanx 1 Tan Square X Quora
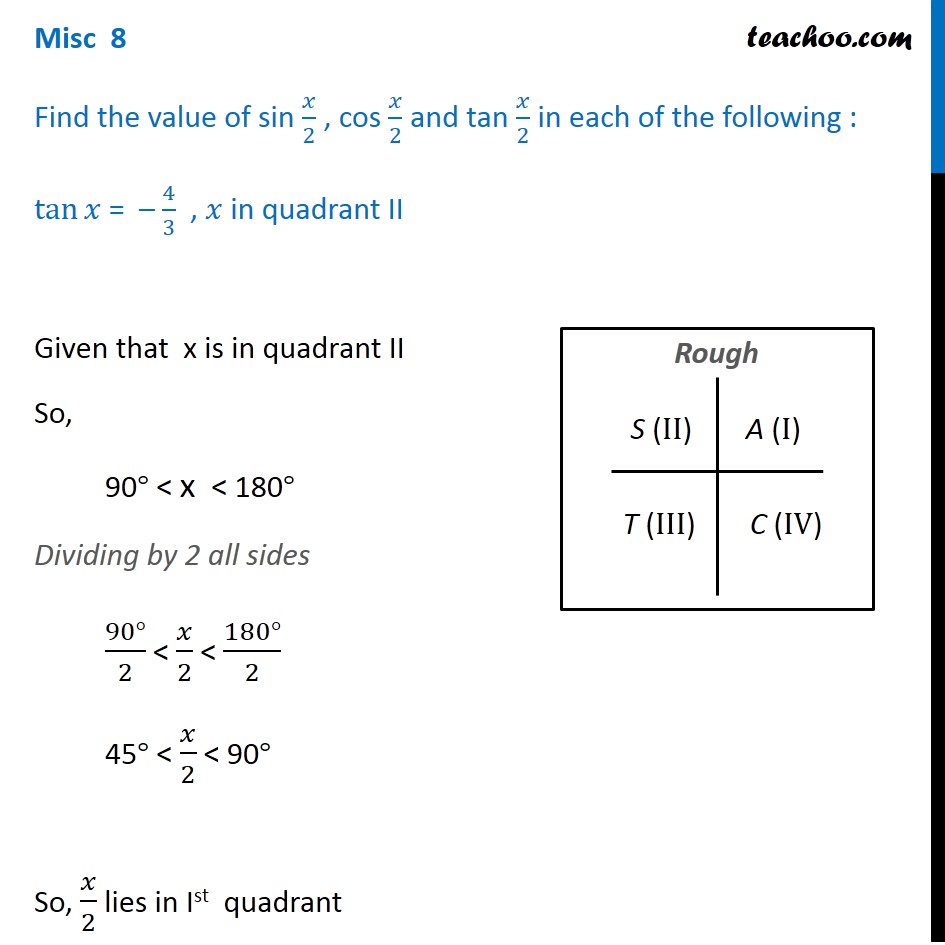



Misc 8 Tan X 4 3 Find Sin X 2 Cos X 2 And Tan X 2
Tan 2x = 2 tan x / (1tan^2x)hence, tan 2x = 12x = pix = pi/2 Two trains leave the station at the same time, one heading west and the other east Lemma 1 cot 2x = (1/2)cot x tan x Proof (1) cot 2x = 1/tan(2x) = cos(2x)/sin(2x) See here for definition of tan (2) cos(2x) = cos 2 (x) sin 2 (x) See Lemma10 Here, $ x $ denotes the greatest integer less than or equal to $ x $ Given that $ f(x) = x x $ The value obtained when this function is integrated with respect to $ x $ with lower limit as $ \frac{3}{2} $ and upper limit as $ \frac{9}{2} $ , is




Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube



Tan2x 1 ただの悪魔の画像
Tan(AB) = tanAtanB/1tanAtanB From this formula we can derive tan(2x) as tan(xx) So tan(2x)= 2tanx/1tanxtanx We can always go for the longer approch from sinx/cosx to 1 Expert Answer 1sin2x = 12sinxcosx = sin^2x cos^2x 2sinxcosx = (sinx cosx)^2 = an alternate way of expressing 1sin2x > if this is what you were looking for What is Tan2x formula?Watch Video in App This browser does not support the video element



Solved Find All Solutions To The Equation Tan 2x 2tanx 1 0 Chegg Com




bestpictjcry Tan 2x Tan 2x
not sure how to start this one, i have tried it a few different ways and i still can't get it (1 tanx)^2 = sec^2x 2tanx int e^ (x) ( (2 tanx)/ (1tanx)cot^ (2) (x (pi)/ (4)))dx is equal to Updated On 299 To keep watching this video solution for FREE, Download our App Join the 2 Crores Student community now!Squaring both sides, we get, tan 4x−2tan 3xtan 2x=1 ⇒tan 4x−2tan 3x−tan 2x2tanx=12tanx−2tan 2x ∴tan 4x−2tan 3x−tan 2x2tanx1=22(tanx−tan 2x)=12=4 using (1) Hence correct option is 'D'



1 Tan 2x Sec 2x
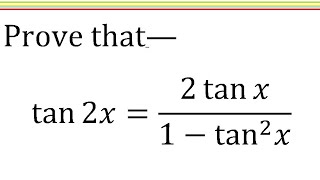



Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube
Sin (b) Cos (a)Cos (b) Therefore, sin (xx) = sin (x)cos (x) cos (x)sin (x) = 2 sin (x) cos (x) Also, Sin 2x = 2 t a n x 1 tan 2 x To Prove Sin2x in the form of tanx x which is equal to 2 t a n x 1 tan 2 x Now let us start the proof from the righthand side and hence, prove it as LHS = RHSYou can put this solution on YOUR website! If(2tanx/(1tan^2x))=1, then x can equal?



1
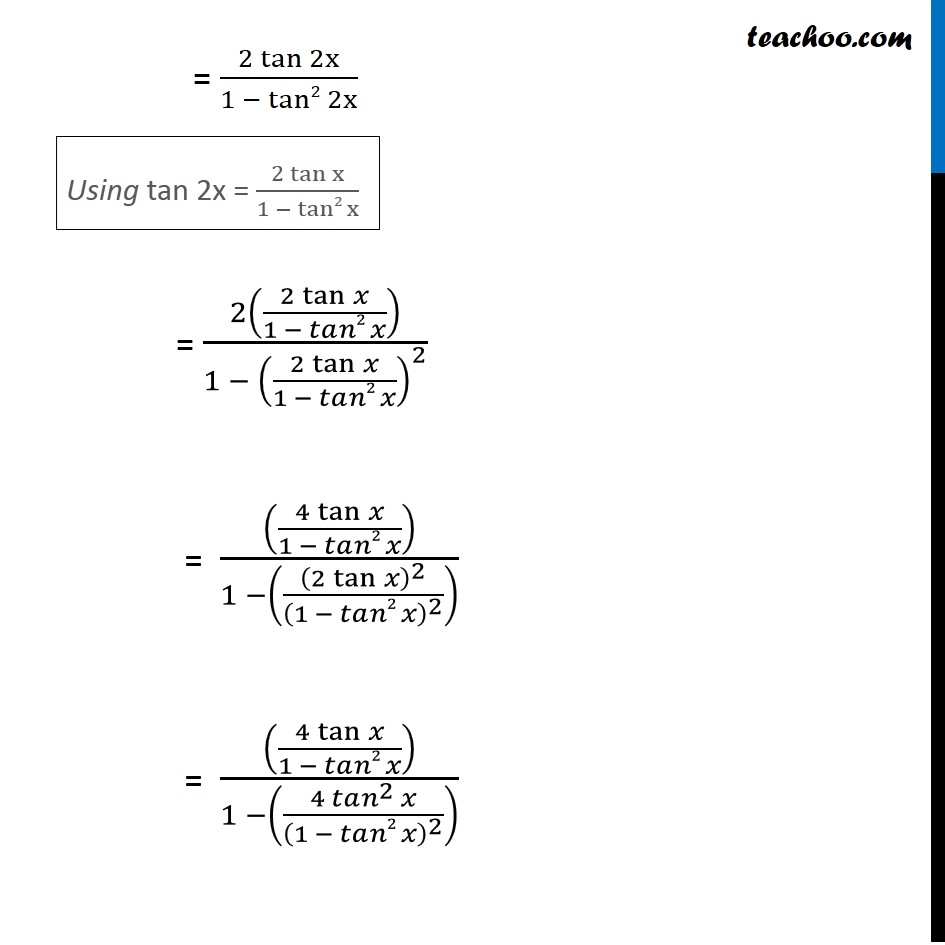



Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x
Explanation 2tanx 1 tan2x = 2 sinx cosx sec2x = 2 sinx cosx 1 cos2x = 2sinx cosx × cos2x 1 = 2sinxcosx = sin2x Answer link Coefficients can be removed when differentiating, ie d/dx 2tanx = 2 d/dx tanx You should know that d/dx tanx = sec2x (either by differentiating sinx/cosx or by just remembering the derivatives of common trig functions, as it will come in handy)Take x common and 1cos2x=2(sin^2 x) hence x tan2x 2tanx/{2(sin^2 x)}^2 is the equation) tan2x = 2tanx/(1 tan^2 x) On substituting and simplifying we get x2tanx tan^2 x / (1 tan^2 x)(4 sin^4 x) Write tanx = sinx/cosx On substituting and simplifying we get 2x / 4sinxcosx (cos^2 x sin^2 x) Write cos^2 x = 1 sin^2 x and take




Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x
.png)



If 2tanx 3tany Then Prove That Tan X Y Homework Help Mycbseguide
Two identities are sin^2x cos^2x = 1 and tan2x=2tanx/1tan^2x calculus find the derivative of the functions F(x)= tan^2(x) and G(x) = sec^2(x) based on your calculations what can you say about F(x) G(x) I found F'x =2 secx^2tanx G'(x)= 2secx^2tanx then when subtracted they equalDo NOT divide by sin bc it can equal zero to find a side, you can divide by sin to find thetaIntroduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formula




Sin2x Sin 2x Identity For Sin2x In Terms Of Tanx Proof Of Sin2x 2tanx 1 Tan 2x Youtube
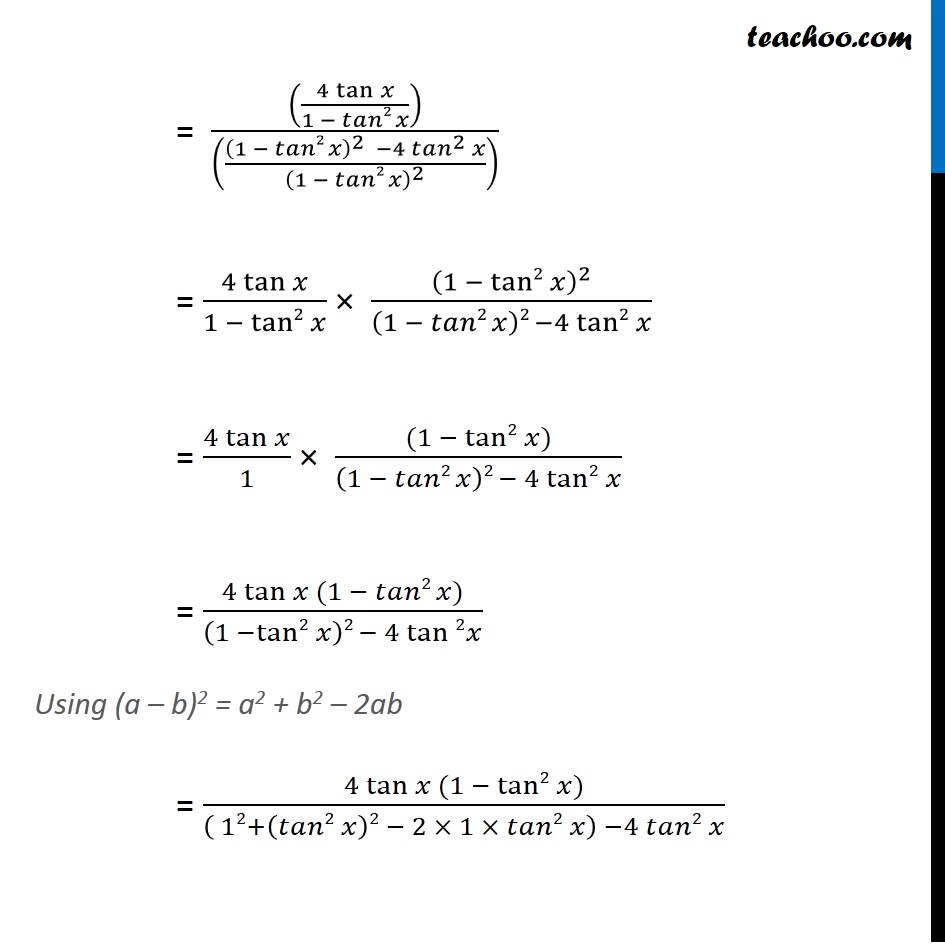



Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x
Use tan x=sinx/cos x, sin 2x = 2 sin x cos x and cos 2x = cos^2xsin^2x, for the right hand side expression 2 tan x/(1tan^2x)=(2sin x/cos x)/(1(sin^2x/cos^2x) =2 sin x cos x/(cos^2xsin^2x) =(sin 2x)/(cos 2x)=tan 2x Proofs for sin 2x = 2 sin x cos x and cos 2x = 1 2 sin^2x Use Area of a triangleABC = 1/2(base)(altitude) = 1/2 bc sin A Here, it is the triangle ABC of a unit Ex 34, 8 Find the general solution of the equation sec2 2x = 1 – tan 2x sec2 2x = 1 – tan 2x 1 tan2 2x = 1 – tan2x tan2 2x tan2x = 1 – 1 tan2 2x tan2x = 0 tan 2x (tan2x 1) = 0 Hence We know that sec2 x = 1 tan2 x So, sec2 2x = 1 tan2 2x tan 2x = 0 taAnswer (1 of 3) There is no difference between (tanx)² and tan²x because we can write (tanx)² = tanx tanx Which is equal to tan²x But there are different in tan²x and tanx² Also difference in ( logx )² and logx² We can write (logx)² as = logx logx But in logx² = 2logx



If Tanx 12 13 Evaluate 2 Sinx Cosx Cos 2x Sin 2x




Answered 2x Given Tan X 2 And Cos X Is Less Bartleby
Basic Trigonometric Function Formulas By using a rightangled triangle as a reference, the trigonometric functions or identities are derived sin θ = Opposite Side/Hypotenuse cos θ = Adjacent Side/Hypotenuse tan θ = Opposite Side/Adjacent Side Shivam Mishra, Meritnation Expert added an answer, on 26/7/16 Shivam Mishra answered this Dear Student, Please find below the solution to the asked query We have f 2 tanx 1 tan 2 x = cos 2 x 1 sec 2 x 2 tanx 2 = cos 2 x 1 2 1 cos 2 x 2 sinx cosx = cos 2 x 1 2 1 2 sinx cosx cos 2 x = cos 2 x 1 2 1 sin 2 x cos 2 x = 2 cos 2 x 1 1 2 1 sin 2 x cos 2 x = 2 cos 2 x 2 1 sin 2 x cos 2 x f 2 tanx 1 tan 2 x = 1 sin 2 x We know that 2 tanx 1 tan The trigonometric equation is sec 2 (x) tan x = 2tan(x) tan x sec 2 (x) 2 = 0 ⇒tan x = 0 and sec 2 (x) 2 = 0 tan x = 0 tan x = tan 0 The genaral solution of tan(θ) = tan(α) is θ = nπ α, where n is an integer ⇒ x = nπ 0 x = nπn = 0,1,2 sec 2 (x) 2 = 0 sec 2 (x) = 2 Using reciprocal identity sec 2 x = 1/cos




Prove That Sin 2x Squared 2tanx Upon 1 Tan Square X Brainly In



What Is The General Solution For Tanthanx Tan 2x Tan 3x Quora
Prove as an identity; Tyrion101 said But is it equal to (2tanx/1tan^2x)^2 is what I'm asking I may have been unclear Yes and no means , which in turn is equal to In what you wrote, you are missing parentheses around the quantity in the denominator, 1 tan 2 (x) What you wrote is the same as #10 symbolipointTrigonometry Solve for x tan (2x)= (2tan (x))/ (1tan (x)^2) tan(2x) = 2tan(x) 1−tan2 (x) tan ( 2 x) = 2 tan ( x) 1 tan 2 ( x) Since x x is on the right side of the equation, switch the sides so it is on the left side of the equation 2tan(x) 1− tan2(x) = tan(2x) 2 tan ( x) 1 tan 2 ( x) = tan ( 2 x)



2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula



Tan2x 1 ただの悪魔の画像
Transcript Ex 76, 24 ∫1 𝑒^𝑥 sec𝑥 (1tan𝑥 )𝑑𝑥 "ex" cos x C (B) "ex" sec x C "ex" sin x C (D) 𝑒𝑥 tan x C ∫1 𝑒^𝑥 sec𝑥 (1tan𝑥 )𝑑𝑥 = ∫1 𝑒^𝑥 (sec𝑥sec𝑥 tan𝑥 )𝑑𝑥 It is of the form ∫1 〖𝑒^𝑥 𝑓(𝑥)𝑓^′ (𝑥) 〗 𝑑𝑥=𝑒^𝑥 𝑓(𝑥)𝐶 Where 𝑓(𝑥)=sec𝑥 𝑓Sin^2xcos^2x=1 tan^2x1=sec^2x cot^2x1=csc^2x Some Pythagorean identities can be rewritten when you prove an identity you are trying to figure out how one side of the equations simplify down to equal the other side of the equation, by doing so you are showing all work 2cos^2x1 tan2x= (2tanx) / (1tan^2x) We will use the following trigonometric formula to prove the formula for tan 2x tan (a b) = (tan a tan b)/ (1 tan a tan b) We have tan 2x = tan (x x) = (tan x tan x)/ (1 tan x tan x) = 2 tan x/ (1 tan 2 x) Hence, we have derived the tan 2x formula using the angle sum formula of the tangent functionWe can prove the double angle identities using the sum formulas for sine and
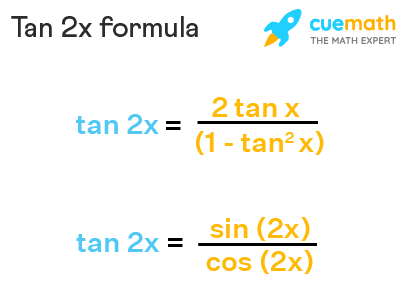



Tan 2x Formula What Is Tan 2x Formula Examples
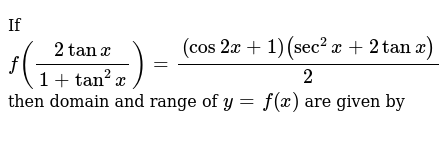



いろいろ Tan2x 1sec2x ただの悪魔の画像
The integral ∫(sec^2x/(secx tanx)^9/2)dx equals (for some arbitrary constant K) asked in Integrals calculus by Rozy ( 418k points) indefinite integrationM1docx Solve(2tanx(1 tan 2 x We have to solve(2tanx(1 tan 2 x Solution(2tanx(1 tan^2x = 21\/tan(x)=tan(x = 2(sin(x cos(x(sin(x)cos(x 2 2 =Please find below the solution to your problem tan x sec x = √3 squaring on both sides (tan x sec x)^2 = √3^2 tan^2x sec^2x 2tanx sec x = 3 sec^2x tan^2x = 1 tan^2x 1 tan^2x 2tanx secx = 3 2tan^2x 2tanxsecx = 31 tan^2x tanx secx = 2/2




Exercise 1 Hy 100 1 X Tw 1 Then Content 17 2 If Y Log Vcosx
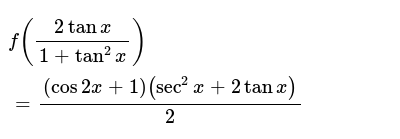



F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2
Using the tan1 x convention may lead to confusion about the difference between arctangent and cotangent It turns out that arctan and cot are really separate things cot(x) = 1/tan(x) , so cotangent is basically the reciprocal of a tangent, or, in Best answer We have f (2tanx/ (1 tan2x)) = 1/2 (1 cos2x) (sec2x tanx) = 1/2x 2cos2x x (1 tan2x 2tanx) = cos2x x (1 tanx)2 = {cosx x (1 tanx)}2 = (cosx sinx)2 = 1 sin (2x) Thus, f (sin 2x) = 1 sin (2x) If sec(xy),sec(x),sec(xy) are in AP then prove that cosx= √2 cos y/2 where cosx and cosy are not equals to 1, Math How do I solve this?




Lim X To 0 2 Tan X 2 Sin X X 2 Sin X Without L Hopital S Rule How Is My Procedure Wrong Mathematics Stack Exchange



What Is The General Solution Of X For Tan X Tan2x 1 Quora
Prove that $\tan^6 °\tan^6 40°\tan^6 80°$ is an integer 1 Find the solution set of the equation $5(\frac{1}{25})^{\sin^2x}45^{\cos2x}=25^{\frac{\sin2x}{2}}$(2tanx / (1 tan 2 x)) = 1 Recall Trigonometry formulas tan2A = (2tanA / (1 tan 2 A)) Substitute (2tanx / (1 tan 2 xIf x = 30°, verify that (i) tan 2x=(2tan x)/(1tan ^2x) CBSE CBSE (English Medium) Class 10 Question Papers 6 Textbook Solutions Important Solutions 3112 Question Bank Solutions 467 Concept Notes & Videos 309 Time Tables 12 Syllabus Advertisement Remove all ads
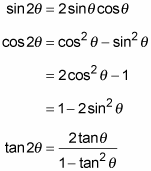



How Do You Prove That Cot2x Tan 2x 1 2tanx Socratic
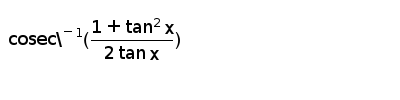



If 2tanx 1 Tan 2x 1 Then X Can Equal
2(sin x / cos x) / 1 – (sin²x / cos²x) = Now tan x = sin x / cos x (2 tan x) / 1 – (sin²x / cos²x) = Remember that tan²x = sin²x / cos²x (2 tan x) / (1 – tan²x) =RHS Hence Proved Alternative method LHS=tan{(2x)} =tan(xx) We know that tan (A B)= tan(A)tan(B) / 1 tan A tan B Applying the same formula we get =>tan(xx)= tan(x)tan(x) / 1 tan x tan x 2tan(x) / (1If (2tanx)/(1tan^2 x)=1/sqrt3, then x can be equal to _____ (check all that apply) a) x=5pi/12 npi b) x=7pi/12 npi c) x=11pi/12 npi d) x=pi/12 npi
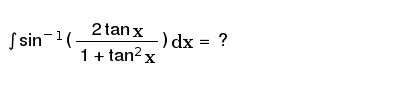



If 2tanx 1 Tan 2x 1 Then X Can Equal



Tan2x ただの悪魔の画像




Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x




If Tan X 2tan 2x 3tan 3x 8cot 8x 3 Then The General Solution Of X




Prove That Tan4x 4tanx 1 Tan 2x 1 6tan X Tan 4x Maths Trigonometric Functions Meritnation Com
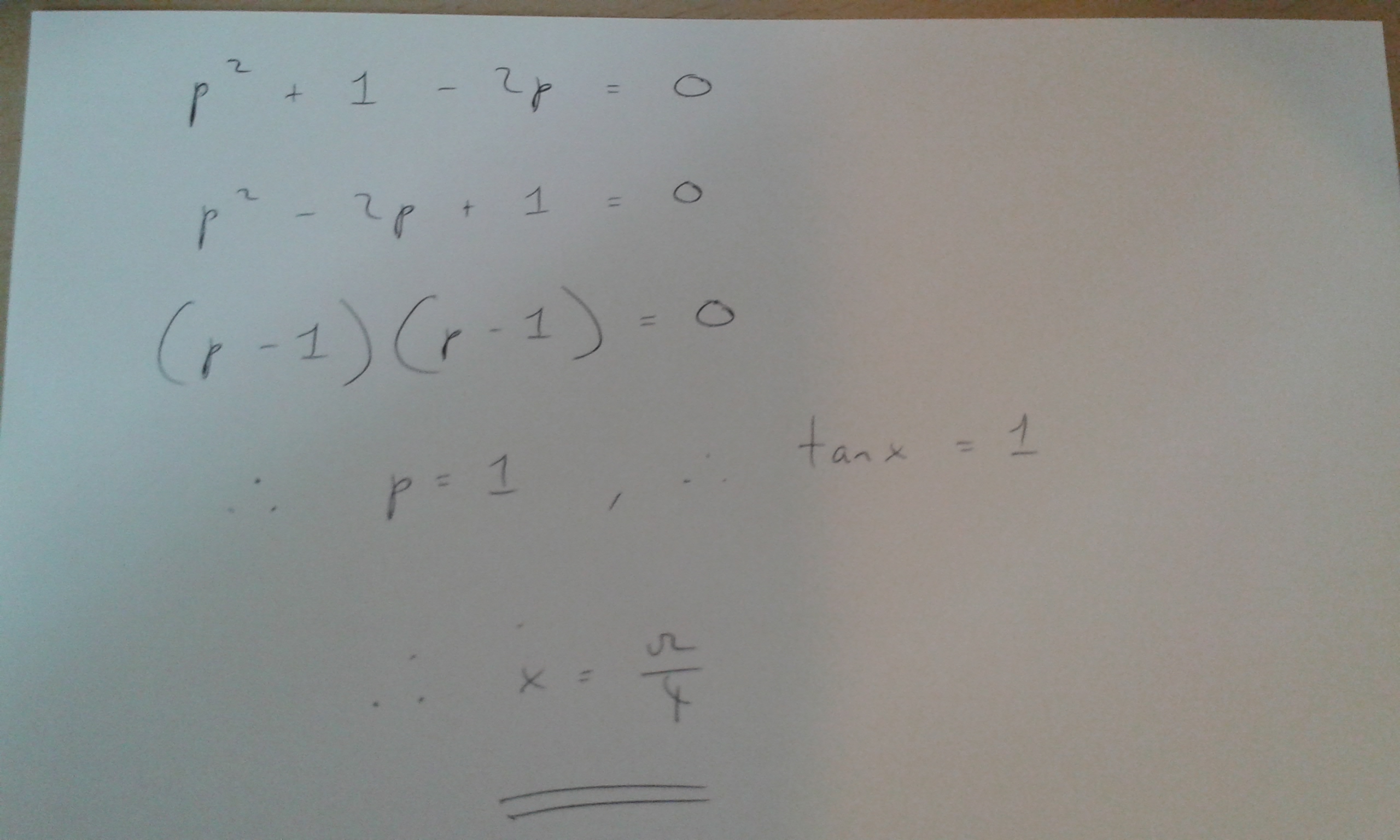



How Do You Solve Sec 2x 2tanx 0 Socratic




How To Take The Derivative Of Tan X Video Lesson Transcript Study Com
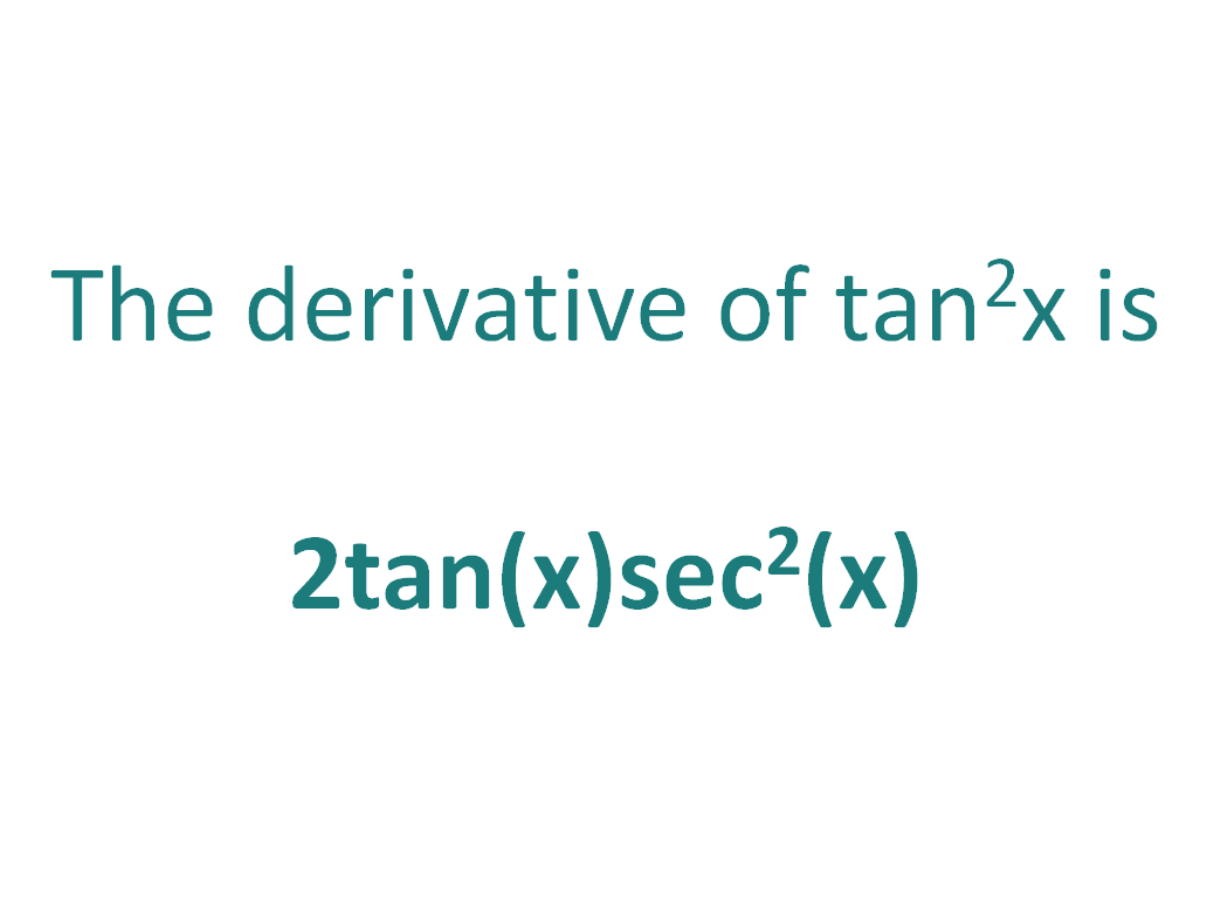



The Derivative Of Tan 2x Derivativeit



Tan2x 1 ただの悪魔の画像




Solved 25 24 7 Complete The Identity 17 Sin X Cos X 2 Chegg Com




Inte X 2tanx 1 Tanx Cot 2 X Pi 4 Dxi Se Q U A Lto E Xtan Pi 4 X C E Xtan X Pi 4 C E Xtan 3pi 4 X C D None Of These




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo




Tan2x 1 ただの悪魔の画像



1




Prove That Sec8x 1 Sec4x 1 Tan8x Tan2x Maths Trigonometric Functions Meritnation Com




Tan2x 1 ただの悪魔の画像




Tangent Half Angle Formula Wikipedia




Sec 2 2x 1 Tan2x Youtube



What Is The Formula Of Tan2x Quora




bestpictjcry Tan 2x Tan 2x




2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula




Which Of The Following Are Solutions To 2tanx 1 Tan 2x Sqrt 3 Check All That Apply Options Are In Brainly Com



Bestpixtajpgjgh If F 2tanx 1 Tan 2x 1 Cos2x Sec 2x 2tanx 2 Then F 4



Fastest What Is Tan 2x Equal To




Tan 2x Formula What Is Tan 2x Formula Examples




Prove That Tan2x 2tanx 1 Tan 2x Youtube




If X 30 Verify That I Tan2x 2tanx 1 Tan 2x Ii Sinx Sqrt 1 Cos2x 2 Youtube



いろいろ Tan2x 1sec2x ただの悪魔の画像




Prove That Tan2x 2tanx 1 Tan X Brainly In
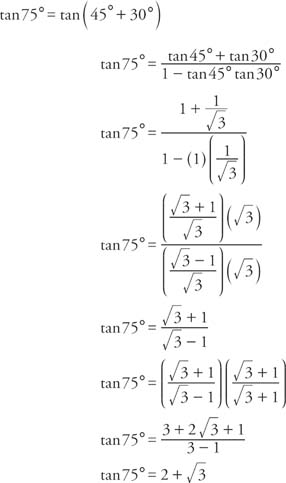



Tangent Identities




Trig Limit 1 Tanx X Pi 4 Compound Angle Substitution Youtube



What Is The Formula Of Tan2x Quora



Evaluate X 0 P 2 Tanx 1 M 2tan 2x Dx Sarthaks Econnect Largest Online Education Community
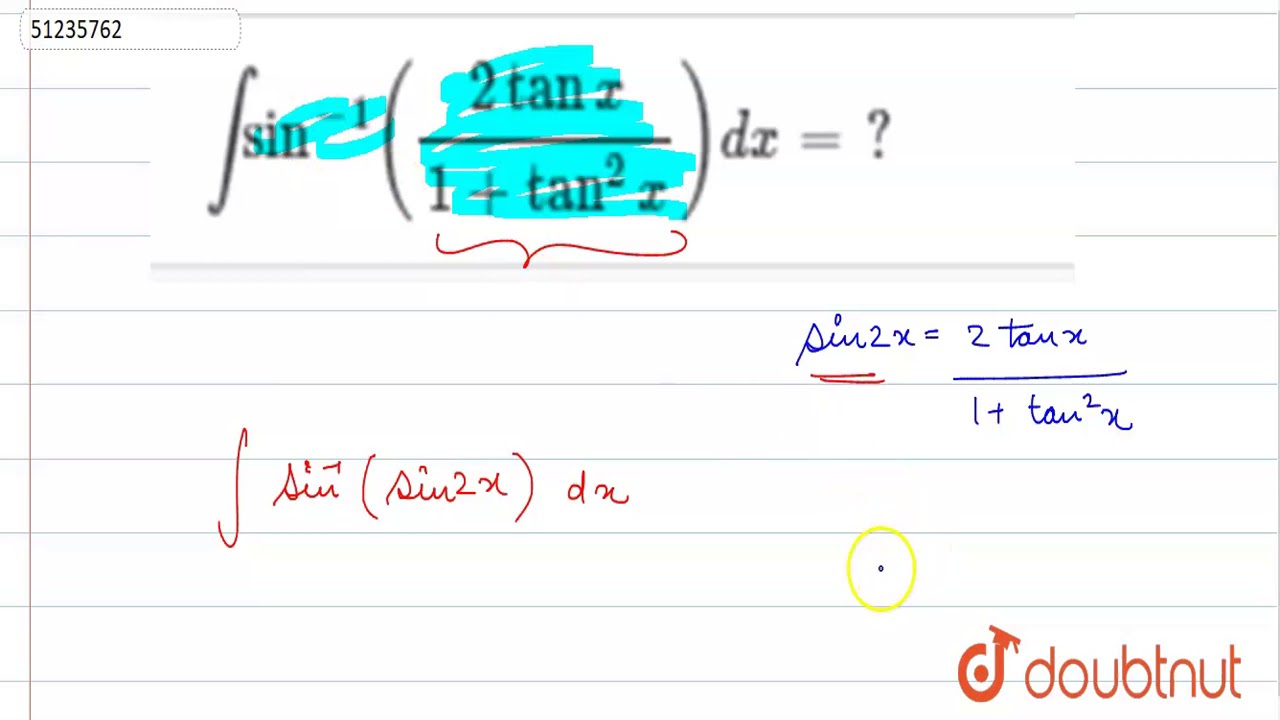



Int Sin 1 2tanx 1 Tan 2 X Dx Youtube



View Question Trigonometric Identities



bestpictjcry Tan 2x Tan 2x




2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula



How To Solve Tan 2x 3tanx 2 0 To Find Tan X Quora



What Is The Formula Of Tan2x Quora



Pinakamabilis Tan 2x Cot 2x 1




If F X Tan 2x Then




1 2tan X 2 1 4 Tanx 4 1 2 X Tan X 2 X




Chapter 7 Trigonometric Identities And Equations Ppt Download




Jpsaepictlqtj Tan 2x Formula Tan 2x Formula In Terms Of Cos X



Fastest What Is Tan 2x Equal To



3




How Do You Solve Sec 2x 2tanx 0 Socratic




1 2tanx Per 1 Tan2x 2 1 Tan2x Per 1 Tan2x Brainly Co Id




Solve Sinxtanx 1 Tanx Sinx




Prove That Tan2x 2tanx 1 Tan 2x Youtube




If X 30 Verify That I Tan2x 2tanx 1 Tan 2x Ii Sinx Sqrt 1 Cos2x 2



Prove That Tan 4x 4tanx 1 Tan 2 X 1 6tan 2 X Tan 4 X Sarthaks Econnect Largest Online Education Community




Evaluate Lim X Tan 1 3 Tan X 2tanx 3 Tan X 4tanx 3
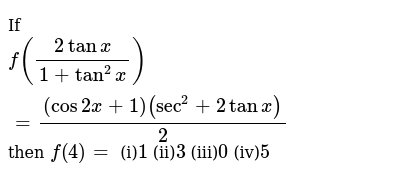



If F 2tanx 1 Tan 2 X Cos2x 1 Sec 2 2tanx 2 Then F 4 I 1 Ii 3 Iii 0 Iv 5




Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco




How Do You Prove 2sin 2x 1 Sinxcosx Tanx Cotx Socratic




Old Version Trig Show Sin 2x 2 Sin X Cos X 2tan X 1 Tan 2 X Youtube
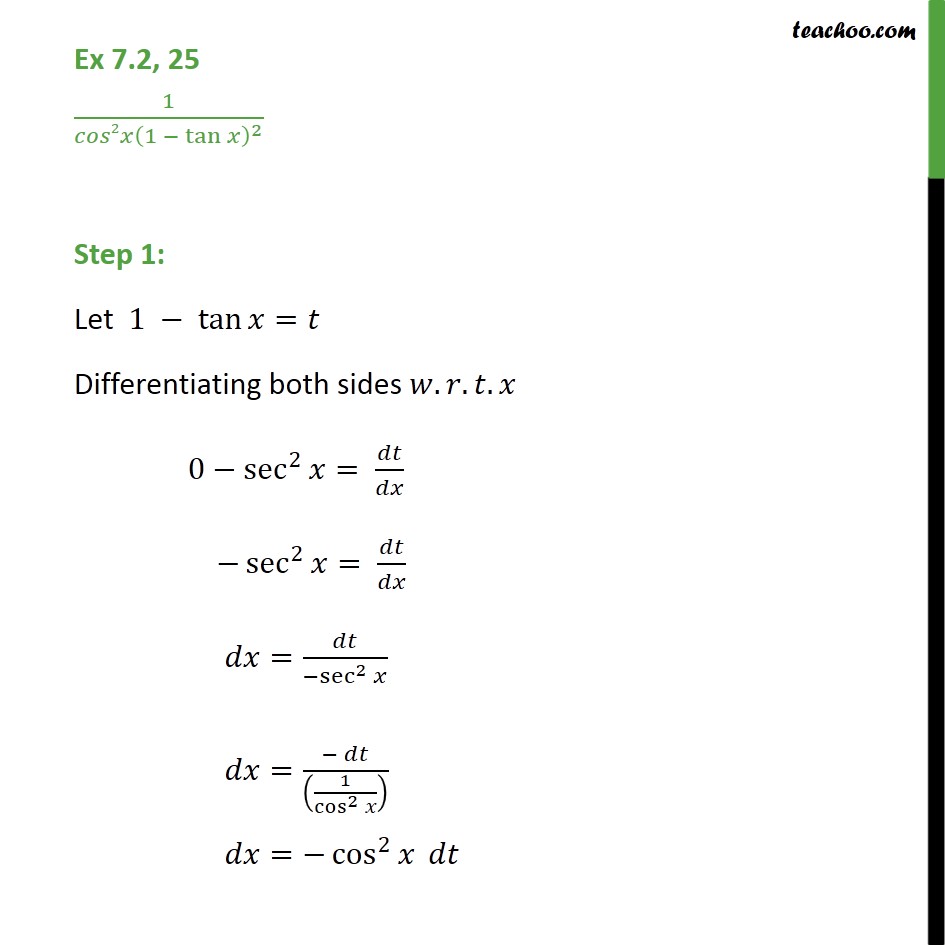



Ex 7 2 25 Integrate 1 Cos 2 X 1 Tan X 2 Ex 7 2



What Is The Formula Of Tan2x Quora



2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula




Analytic Trig Ppt Video Online Download




Limit X Tends P 4 Sec 2x 2 Tanx 1 Brainly In
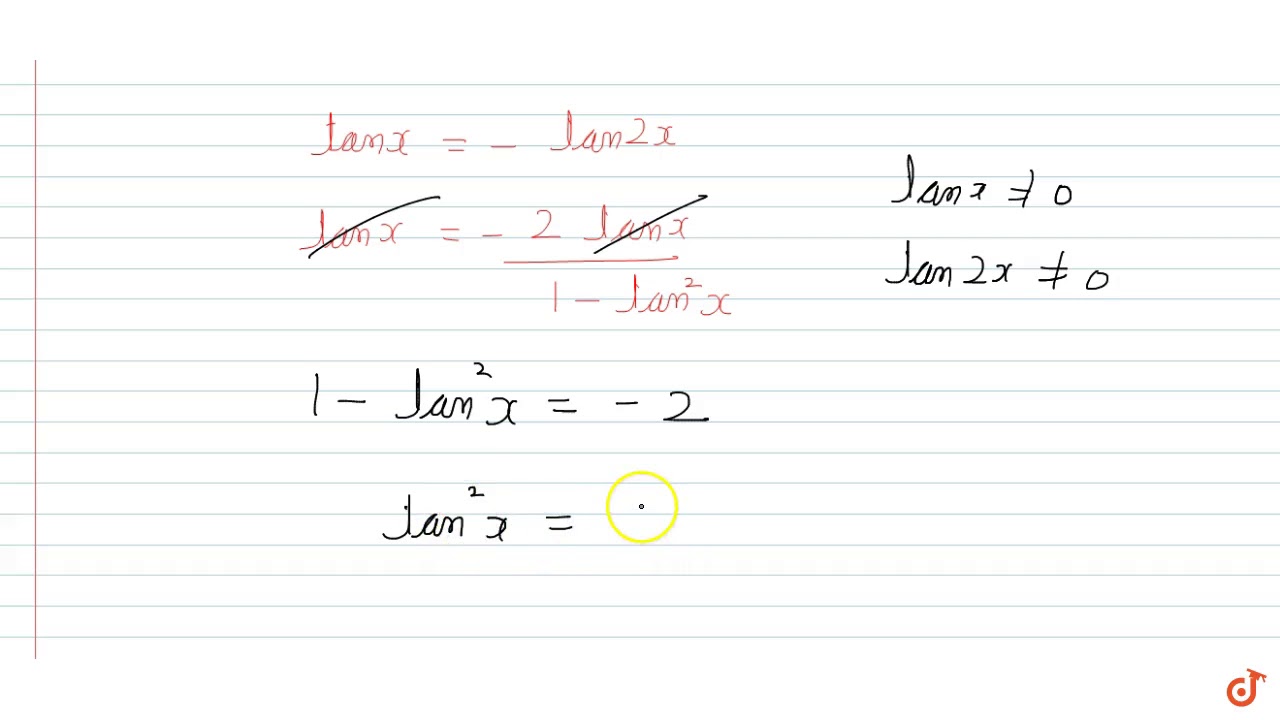



The General Solution Of The Equation Tanx Tan 2x Tan 2x Tanx 2 0 Is Youtube



The Solution Of Differential Equation Dy Dx Y Tanx 2x X 2tanx Is Sarthaks Econnect Largest Online Education Community



Tan2x 2tanx 1 Tan X Trigonometric Identity Solve Hindi Youtube




The Nearest Degree Tan 2x 2tanx 0 In The Interval 0 X 3pi 2 A 63 180 243 360b 0 63 180 243 360c 63 243d 0 180 360 Study Com
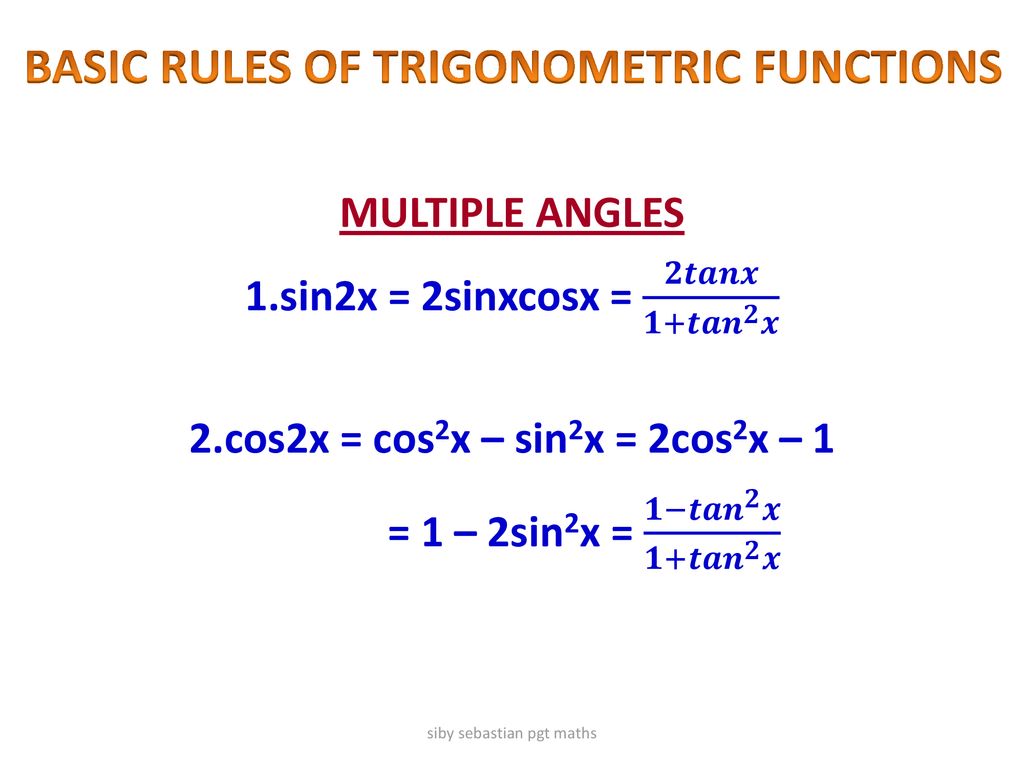



By Siby Sebastian Pgt Maths Ppt Download




Derivative Of Tan X Old Video Khan Academy




How Do You Prove Tan 2x Secx 1 1 Secx Socratic




Fastest What Is Tan 2x Equal To



Prove Sin2x 2tanx 1 Tan 2x Socratic



What Is The Formula Of Tan2x Quora




2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula
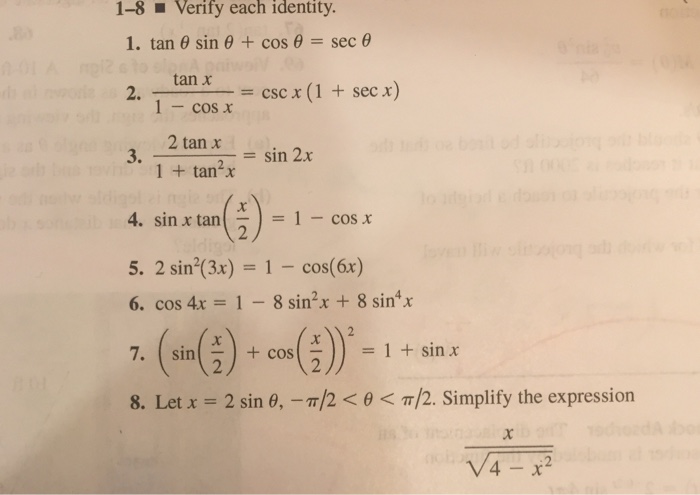



2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula



Solve Cot 2x 0 The Student Room




2tanx Cotx 1 0 Youtube



0 件のコメント:
コメントを投稿